Integration Formulas For Class 12: The Integration formula is a mathematical expression representing the process of finding the antiderivative of a function. It provides a systematic way to evaluate definite or indefinite integrals. Integration formulas are also known as integral formulas or antiderivative formulas.
In calculus, integration is the reverse process of differentiation. It involves finding the antiderivative of a function, which is another function whose derivative is equal to the original function. The Integration formulas provide a set of rules and patterns that help simplify the process of finding antiderivatives. Mathematicians and scientists can use these formulas to solve a variety of integration-related problems because they cover a wide range of functions.
This article seeks to offer a thorough grasp of integration formulas, including lists of differentiation and integration formulas, basic integration formula listings, and access to the Integration Formulas PDF from the mathematics portal, along with additional information on integration formulas.
What Is Integration Formulas?
Integration formulas play a fundamental role in calculus, providing a systematic approach to finding antiderivatives of functions. Understanding integration as a concept is crucial to understanding the value of the integration formula. Integration is the process of finding the antiderivatives of a function. It involves ‘undoing’ the differentiation process and recovering the original function from its derivative. The indefinite integral, denoted as ∫f(x) dx, represents the family of functions that have the derivative f(x).
In calculus, integration is the reverse process of differentiation. Given a function f(x), its antiderivative F(x) is a function whose derivative is equal to f(x), ie., F′(x) = f(x). The integral formula allows us to find F(x) given f(x).
The integral formula is represented by the symbol ∫ (the integral symbol), followed by the function to be integrated, and then dx (or another variable of integration) to indicate the variable with respect to which the integration is performed.
For example, the integral formula for finding the antiderivative of a function f(x) is expressed as ∫ f(x) dx = F(x) + C. Here, F(x) represents the antiderivative of f(x), and C is the constant of integration. The constant of integration arises because when we integrate a function, there can be infinitely many antiderivatives that differ by a constant value.
An Integration formula covers a wide range of rules and techniques for integrating different types of functions, such as polynomials, exponential functions, trigonometric functions, logarithmic functions, and more. In calculus, physics, engineering, and other analyze mathematical models, these formulas provide a systematic method for evaluating integrals and are essential tools.
Integration Formulas For Class 12:
Students usually learn about integral calculus, including various integration techniques and formulas in class 12 mathematics. Here are some commonly taught integration formulas in Class 12.
- Constant Rule: ∫ k dx = kx + C, Integration of a constant term, where k is a constant and C is the constant of integration.
- Power Rule: ∫ x^n dx = (x^(n+1))/(n+1) + C, Integration of a power of x, where n is any real number except -1.
- Reciprocal Rule: ∫ (1/x) dx = In (x) + C, Integration of the reciprocal of x.
- Exponential Rule: ∫ e^x dx = e^x + C, Integration of the exponential function e^x.
- Sum and Difference Rules: ∫ (f(x) ± g(x)) dx = ∫ f(x) dx ± ∫ g(x) dx.
- Constant Multiple Rule: ∫ C * f(x) dx = C * ∫ f(x) dx.
- Trigonometric Integration Formulas:
- ∫ sin(x) dx = -cos(x) + C, Integration of the sine function.
- ∫ cos(x) dx = sin(x) + C, Integration of the cosine function.
- ∫ sec^2(x) dx = tan(x) + C, Integration of the secant squared function.
- ∫ csc^2(x) dx = -cot(x) + C, Integration of the cosecant squared function.
- ∫ sec(x) tan(x) dx = sec(x) + C and ∫ cosec(x) cot(x) dx = -cosec (x) + C.
- Integration by Substitution: ∫ f(g(x))* g′(x) dx = ∫ f(u) du, Integration using the substitution method, where u = g(x).
- Integration by Parts: ∫ u dv = uv-∫ v du, Integration using the product rule, where u and v are functions of x.
- Trigonometric Substitution: ∫ (a^2 – x^2) ^ (½) dx = a ∫ sec^2(θ) dθ, where x = a sin(θ) or x = a tan(θ).
- Partial Fraction Decomposition: ∫ (f(x) / (ax+b)) dx = Inㅣax+bㅣ+ C. C is the constant of integration.
Differentiation And Integration Formulas:
The following are the general formulas used in Differentiation and Integration.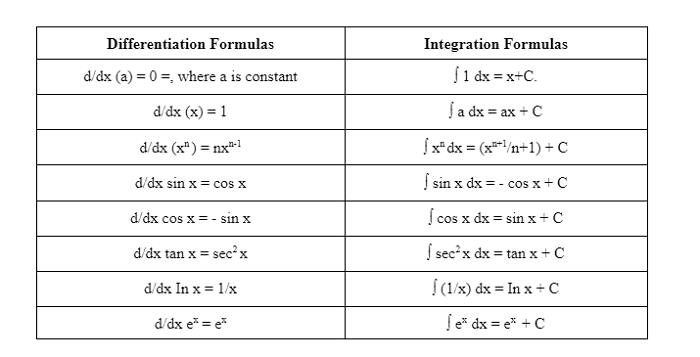
Basic Integration Formulas List:
The following is the basic Integration Formula List.

How To Download Integration Formulas List In PDF Format?
Students can also download the all integration formulas pdf from the math portal. The following are the steps to download the Integration Formula PDF.
- The student should visit the math portal at https://www.mathportal.org.
- The Math Portal home page will appear on the screen. Here go to the “Math Formulas” tab on the navigation bar and click on it.
- Now, scroll down the Math Formula page and click on the “Download Integration Formula PDF” link under the “Integration Formula” section.
- The Integration formula pdf file will appear on the screen. Now, click on the “Download ⤓” symbol to download the file.
- Or you can directly click on the https://www.mathportal.org/ link and download the Integration Formula PDF file
- In this way, you can easily download the Integration formula pdf file from the Math Portal.
An integration Formula is a powerful tool in calculus, providing a systematic way to find antiderivatives of functions. Understanding these rules and the techniques for integration allows mathematicians and scientists to solve a wide range of problems across various disciplines. One can develop a strong foundation in integration and confidently apply it to real-world scenarios by investigating fundamental formulas, sophisticated methodologies, and specific cases.